Use the Law of Cosines to solve the triangles. Round lengths to the nearest tenth and angle measures to the
nearest degree. PLEASE solve both of these triangles. Thank you!
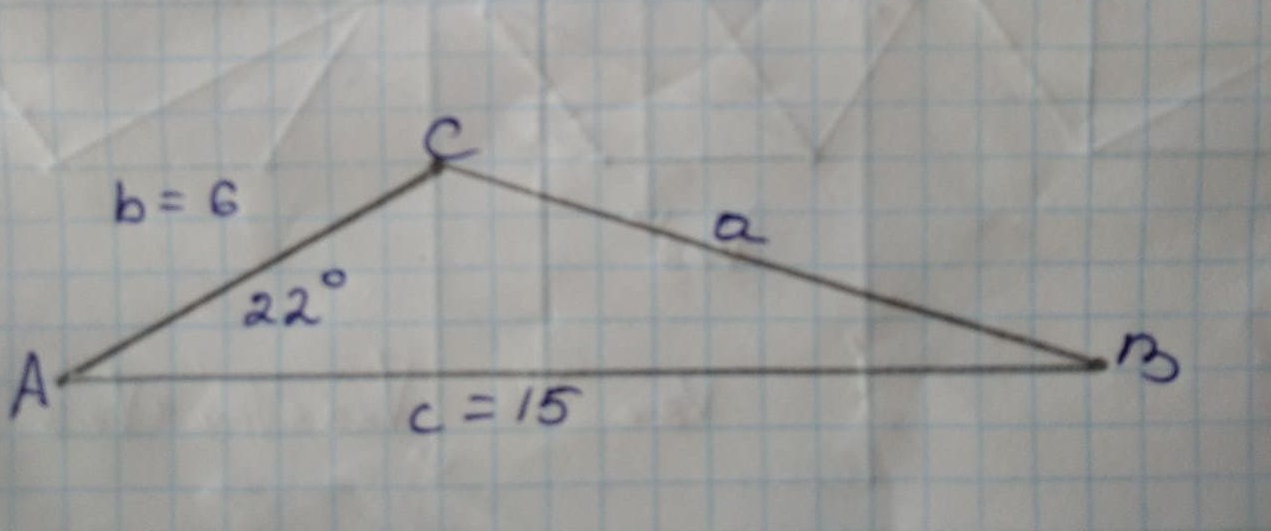

1 Answers
Best Answer
using Cosine rule, cosA =(b^(2)+c^(2)-a^(2))/(2bc)
-> cos(22)=((6)^(2)+(15)^(2)-a^(2))/(2(6)(15))
-> 0/9272=(36+225-a^(2))/(180)
-> 166.893=261-a^(2)
-> a^(2)=94.1069
-> a=9.7009
-> ~ 10
using Sine rule, (sin A)/(a)=(sinB)/(b)
-> (sin(22))/(10)=(sinB)/(6)
-> sin B=(6sin(22))/(10)
-> B~ 13°
using angle sum property of a triangle,
C = 180- (A+B)
= 180 - (22 + 13)
= 145°
Therefore, a=10,B=13°,C=145°

